
It should be recognized that it is impossible to determine with certainty any date prior to the beginning of historical records—except, of course, by divine revelation. Science, in the proper sense, is based on observation, and we have no records of observation except historical records. Natural processes can be used to estimate prehistoric dates, but not to determine such dates. The accuracy of the estimates will depend on the validity of the assumptions applied to the use of the processes in making such calculations. Assume, in the general case, a simple process in which there are two main components, one "parent" and one "daughter" component—call them A and B, respectively. The initial magnitudes of these components at zero time (that is, the time when the particular system came into existence) are A0 and B0. After an additional time T these magnitudes have changed to AT and BT. The average time-rate at which A changes into B during the time T is RT. The instantaneous rate may either be constant or may change in some fashion with time, in which case it may be expressed in functional form as: (1)
Jump Back to Lower Text rt = f (A0, B0, t), since it may possibly depend on the process components as well as on time. If the process is not a closed system, then there may be changes in A and B which result from extraneous influences, other than those expressed in the normal rate function. Let such changes be represented by the quantities Da and Db where Da may be either positive or negative and represents the modification in A brought about during the time T by such external influences. A similar definition applies to Db. Putting all these quantities together, the following equations express the effect of these changes in A and B. (2) A0 ± Da - (RT)T= AT (3) B0 ± Db + (RT)T = BT Subtracting equation (3) from equation (2): (4) (A0 - B0) ± (D a ± D b) - 2RT(T) = (AT - BT) from which the time T is calculated as follows: (5)
Jump Back to Lower Text | T = | (BT - B0) + (A0 - AT) ± (D a ±D b) | | 2RT | This equation is relatively simple, involving only two components in the chronometric system. Many processes would involve more than this. Some, of course, might involve a change in only one component. To solve the equation and obtain the duration T, it is obvious that all the terms on the right-hand side of equation (5) would have to be known. The problem, however, is that only AT, BT, and rT (the present magnitudes and rate) can actually be measured. There is no way by which the average rate RT can be determined unless the functional relationship expressed in equation (1) is known. Mathematically this average rate could be expressed as follows: (6)
Jump Back to Lower Text 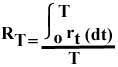 This cannot be calculated, however, unless the equation
for rt is known. It is customary simply to assume that RT = (rt) as it is measured at present. In other words, it is arbitrarily assumed that the process rate has been constant throughout the period T. This is an unrealistic assumption since, in the real world, there is no such thing as a process rate which cannot be changed. Furthermore, there is no way by which D a and D b can be determined, since there is no way of knowing what extraneous influences may have affected the system in the prehistoric past. The common assumption is that the system has always been a closed system and thus both D a and D b are zero, but this assumption is likewise unrealistic since, in the real world, all systems are open systems. Similarly, there is no way of knowing the initial magnitudes of the parent and daughter components, A0 and B0, since no scientific observers were present to measure them at the time. Again, however, it is commonly assumed that there was no daughter component present initially, so that BO is zero, and that the initial parent component has been modified only by the amount corresponding to the present daughter component, so that A0 = BT + AT.
If all these assumptions are made, then equation (5) becomes: (7)
Jump Back to Lower Text | T = | (BT - 0) + (BT + AT - AT) + (0+0) | = | BT | | 2RT | RT | Since both BT and rT can be measured, it is thus easily possible to calculate T. However, the resulting date is obviously only as accurate as the assumptions. To recapitulate, any geochronometric calculation is based on at least the following assumptions: 1. Constant process rate (or known functional variation of process rate). 2. Closed process system (or known external effects on the open system). 3. Initial process components known. It is significant that not one of these three vital assumptions is provable, or testable, or reasonable, or even possible! Therefore, no geochronometric calculation can possibly be certain, and most of them are bound to be vastly in error. Since the magnitude of the error in the assumptions obviously will vary quite widely from process to process, one would expect to get a wide range of "apparent ages" from different processes. In Table I have been listed 76 different processes for calculating the age of various integral parts of the earth and, thus, presumably of the earth itself. All of them yield an age of much less than a billion years, whereas the present standard evolutionary estimate is approximately five billion years. The presently-favored geochronometric methods (that is, those that give long ages, such as uranium-lead, rubidium-strontium, and potassium-argon) have not been included in the tabulation, nor are they discussed in this paper. However, it has been shown elsewhere (1, 5, 6, 7) that these can also easily be reconciled with young-age concepts. The most obvious characteristic of the values listed in the table is their extreme variability—all the way from 100 years to 500,000,000 years. This variability, of course, simply reflects the errors in the fundamental uniformitarian assumptions. Nevertheless, all things considered, it seems that those ages on the low end of the spectrum are likely to be more accurate than those on the high end. This conclusion follows from the obvious fact that: (1) they are less likely to have been affected by initial concentrations or positions other than "zero"; (2) the assumption that the system was a "closed system" is more likely to be valid for a short time than for a long time; (3) the assumption that the process rate was constant is also more likely to be valid for a short time than for a long time. Thus, it is concluded that the weight of all the scientific evidence favors the view that the earth is quite young, far too young for life and man to have arisen by an evolutionary process. The origin of all things by special creation—already necessitated by many other scientific considerations—is therefore also indicated by chronometric data. Finally, the reader should note that these conclusions were reached with no reference at all to the testimony of the Bible relative to chronology. It is, therefore, all the more significant that these results correspond closely to the brief chronology of terrestrial and human history given long ago by divine revelation in the Holy Scriptures. TABLE I
Uniformitarian Estimates—Age of the Earth
(Unless otherwise noted, based on standard assumptions of closed systems, constant rates, and no initial daughter components.) | | Process | Indicated Age of Earth | Reference | | 1. | Efflux of Helium-4 into the atmosphere | 1,750 - 175,000 years | [1] | | 2. | Influx of meteoritic dust from space | too small to calculate | 1 | | 3. | Influx of radiocarbon to the earth system | 5,000 - 10,000 years | 1 | | 4. | Development of total human population | less than 4,000 years | 1 | | 5. | Influx of uranium to the ocean via rivers | 10,000 - 100,000 years | 1 | | 6. | Influx of sodium to the ocean via rivers | 260,000,000 years | 1 | | 7. | Influx of nickel to the ocean via rivers | 9,000 years | 1 | | 8. | Influx of magnesium to the ocean via rivers | 45,000,000 years | 1 | | 9. | Influx of silicon to the ocean via rivers | 8,000 years | 1 | | 10. | Influx of potassium to the ocean via rivers | 11,000,000 years | 1 | | 11. | Influx of copper to the ocean via rivers | 50,000 years | 1 | | 12. | Influx of gold to the ocean via rivers | 560,000 years | 1 | | 13. | Influx of silver to the ocean via rivers | 2,100,000 years | 1 | | 14. | Influx of mercury to the ocean via rivers | 42,000 years | 1 | | 15. | Influx of lead to the ocean via rivers | 2,000 years | 1 | | 16. | Influx of tin to the ocean via rivers | 100,000 years | 1 | | 17. | Influx of aluminum to the ocean via rivers | 100 years | 1 | | 18. | Influx of carbonate to the ocean via rivers | 100,000 years | [2] | | 19. | Influx of sulphate to the ocean via rivers | 10,000,000 years | 2 | | 20. | Influx of chlorine to the ocean via rivers | 164,000,000 years | 2 | | 21. | Influx of calcium to the ocean via rivers | 1,000,000 years | 2 | | 22. | Leaching of sodium from continents | 32,000,000 years | 2 | | 23. | Leaching of chlorine from continents | 1,000,000 years | 2 | | 24. | Leaching of calcium from continents | 12,000,000 years | 2 | | 25. | Influx of sediment to the ocean via rivers | 30,000,000 years | [3] | | 26. | Erosion of sediment from continents | 14,000,000 years | 3 | | 27. | Decay of earth's magnetic field | 10,000 years | [4] | | 28. | Efflux of oil from traps by fluid pressure | 10,000 - 100,000 years | [5] | | 29. | Formation of radiogenic lead by neutron capture | too small to measure | 5 | | 30. | Formation of radiogenic strontium by neutron capture | too small to measure | 5 | | 31. | Decay of natural remanent paleomagnetism | 100,000 years | 5 | | 32. | Decay of C- 14 in pre-Cambrian wood | 4,000 years | 5 | | 33. | Decay of uranium with initial lead | too small to measure | [6] | | 34. | Decay of potassium with entrapped argon | too small to measure | 6 | | 35. | Influx of juvenile water to oceans | 340,000,000 years | [7] | | 36. | Influx of magma from mantle to form crust | 500,000,000 years | 7 | | 37. | Growth of active coral reefs | 10,000 years | 7 | | 38. | Growth of oldest living part of biosphere | 5,000 years | 7 | | 39. | Origin of human civilizations | 5,000 years | 7 | | 40. | Formation of river deltas | 5,000 years | [8] | | 41. | Submarine oil seepage into oceans | 50,000,000 years | [9] | | 42. | Decay of natural plutonium | 80,000,000 years | [10] | | 43. | Decay of lines of galaxies | 10,000,000 years | [11] | | 44. | Expanding interstellar gas | 60,000,000 years | [12] | | 45. | Formation of Carbon 14 on meteorites | 100,000 years | [13] | | 46. | Decay of short-period comets | 10,000 years | [14] | | 47. | Decay of long-period comets | 1,000,000 years | [15] | | 48. | Influx of small particles to the sun | 83,000 years | 15 | | 49. | Maximum life of meteor showers | 5,000,000 years | 15 | | 50. | Accumulation of dust on the moon | 200,000 years | 15 | | 51. | Deceleration of earth by tidal friction | 500,000,000 years | [16] | | 52. | Cooling of earth by heat efflux | 24,000,000 years | 16 | | 53. | Accumulation of calcareous ooze on sea floor | 5,000,000 years | [17] | | 54. | Influx of lithium into ocean via rivers | 20,000,000 years | [18] | | 55. | Influx of titanium into ocean via rivers | 160 years | 18 | | 56. | Influx of chromium into ocean via rivers | 350 years | 18 | | 57. | Influx of manganese into ocean via rivers | 1,400 years | 18 | | 58. | Influx of iron into ocean via rivers | 140 years | 18 | | 59. | Influx of cobalt into ocean via rivers | 18,000 years | 18 | | 60. | Influx of zinc into ocean via rivers | 180,000 years | 18 | | 61. | Influx of rubidium into ocean via rivers | 270,000 years | 18 | | 62. | Influx of strontium into ocean via rivers | 19,000,000 years | 18 | | 63. | Influx of bismuth into ocean via rivers | 45,000 years | 18 | | 64. | Influx of thorium into ocean via rivers | 350 years | 18 | | 65. | Influx of antimony into ocean via rivers | 350,000 years | 18 | | 66. | Influx of tungsten into ocean via rivers | 1,000 years | 18 | | 67. | Influx of barium into ocean via rivers | 84,000 years | 18 | | 68. | Influx of molybdenum into ocean via rivers | 500,000 years | 18 | | 69. | Influx of bicarbonate into ocean via rivers | 700,000 years | [19] | | 70. | Escape of high-velocity stars from globular clusters | 40,000 years | [20] | | 71. | Rotation of spiral galaxies | 200,000,000 years | 20 | | 72. | Accumulation of peat in peat bogs | 8,000 years | [21] | | 73. | Accumulation of sediments for sedimentary rocks | 20,000 years | 21 | | 74. | Lithification of sediments to form sedimentary rocks | 20,000 years | 21 | | 75. | Instability of rings of Saturn | 1,000,000 years | 15 | | 76. | Escape of methane from Titan | 20,000,000 years | 15 |

|
References
1. Henry M. Morris (Ed.), Scientific Creationism for Public Schools (San Diego, Institute for Creation Research, 1974). Return to Text (first occurance)
2. Dudley J. Whitney, The Face of the Deep (New York, Vantage Press, 1955). Return to Text (first occurance)
3. Stuart E. Nevins, "Evolution: The Ocean Says No.", Impact Series, ICR Acts and Facts, Vol. 2, No. 8., October 1973. Return to Text (first occurance)
4. Thomas G. Barnes, Origin and Destiny of the Earth's Magnetic Field (San Diego, Institute for Creation Research, 1973). Return to Text
5. Melvin A. Cook, Prehistory and Earth Models (London, Max Parrish, 1966). Return to Text (first occurance)
6. Harold S. Slusher, Critique of Radiometric Dating (San Diego, Institute for Creation Research, 1973). Return to Text (first occurance)
7. John C. Whitcomb, Jr., and Henry M. Morris, The Genesis Flood (Philadelphia, Presbyterian and Reformed, 1961). Return to Text (first occurance)
8. Benjamin F. Allen, "The Geologic Age of the Mississippi River", Creation Research Society Quarterly, Vol. 9 (September 1972), pp. 96-114. Return to Text
9. R. D. Wilson et al., "Natural Marine Oil Seepage", Science (Vol. 184), May 24, 1974, pp. 857-865. Return to Text
10. "Natural Plutonium", Chemical and Engineering News, September 20, 1971. Return to Text
11. Halton Arp, "Observational Paradoxes in Extragalactic Astronomy", Science, Vol. 174 (December 17, 1971), pp. 1189-1200. Return to Text
12. V. A. Hughes and D. Routledge, "An Expanding Ring of Interstellar Gas with Center Close to the Sun", Astronomical Journal, Vol. 77. No. 3, (1972) pp. 210-214 Return to Text
13. R. S. Boekl, "Search for Carbon 14 in Tektites", Journal of Geophysical Research, Vol. 77, No. 2 (1972), pp. 367-368. Return to Text
14. Harold S. Slusher, "Some Astronomical Evidences for a Youthful Solar System", Creation Research Society Quarterly, Vol. 8 (June 1971), pp. 55-57. Return to Text
15. Harold S. Slusher, "Age of the Earth from some Astronomical Indicators", Unpublished manuscript. Return to Text (first occurance)
16. Thomas G. Barnes, "Physics, A Challenge to Geologic Time", Impact Series 16, ICR Acts and Facts, Institute for Creation Research, July 1974. Return to Text (first occurance)
17. Maurice Ewing, J. I. Ewing & M. Talwan, "Sediment Distribution in the Oceans-Mid-Atlantic Ridge", Bulletin of the Geological Society of America, Vol. 75 (January 1964), pp. 17-36. Return to Text
18. Chemical Oceanography, Ed. by J. P. Riley and G. Skirrow (New York, Academic Press, Vol. 1, 1965), p. 164. See also Harold Camping, "Let the Oceans Speak", Creation Research Society Quarterly, Vol. 11, (June 1974), pp. 39-45. Return to Text (first occurance)
19. Stuart E. Nevins, "How Old is the Ocean?", Unpublished manuscript. Return to Text
20. George Mulfinger, "Critique of Stellar Evolution", Creation Research Society Quarterly, Vol. 7 (June 1970), pp. 7-24. Return to Text (first occurance)
21. Henry M. Morris, Unpublished calculations. Return to Text (first occurance)
 |

